(1-tan^2(x/2))/(1+tan^2(x/2)) を解くのに必要な道具
※数式がスマホで画面からはみ出る場合、横スクロールするかピンチインしてください。
目次
\(\frac{1-tan^2\frac{x}{2}}{1+tan^2\frac{x}{2}}\)を解くのに必要な道具 (1-tan^2(x/2))/(1+tan^2(x/2)) を解くのに必要な道具
cosの倍角の公式
$$cos2\theta=2cos^2\theta-1$$
cosの倍角の公式の証明
cosの加法定理cos(α+β)=cosαcosβ-sinαsinβにおいてα=β=θとおくと
$$cos2\theta=cos^2\theta-sin^2\theta=cos^2\theta-(1-cos^2\theta)=2cos^2\theta-1$$
tanの倍角の公式
$$tan2\theta=\frac{2tan\theta}{1-tan^2\theta}$$
tanの倍角の公式の証明
tanの加法定理tan(α+β)=\(\frac{tan\alpha+tan\beta}{1-tan\alpha\ tan\beta}\)においてα=β=θとおくと
$$tan2\theta=\frac{tan\theta+tan\theta}{1-tan\theta\ tan\theta}=\frac{2tan\theta}{1-tan^2\theta}$$
\(\frac{1-tan^2\frac{x}{2}}{1+tan^2\frac{x}{2}}\)の解法 (1-tan^2(x/2))/(1+tan^2(x/2)) の解法
\(tan\frac{x}{2}\)=tとおくと
$$\frac{1-tan^2\frac{x}{2}}{1+tan^2\frac{x}{2}}=\frac{1-t^2}{1+t^2}=\frac{2}{1+t^2}-1=2\left(\frac{1}{1+t^2}\right)-1$$
tを\(tan\frac{x}{2}\)に戻すと
$$2\left(\frac{1}{1+t^2}\right)-1=2\left(\frac{1}{1+tan^2\frac{x}{2}}\right)-1=2cos^2\frac{x}{2}-1$$
cosの倍角の公式cos2θ=\(2cos^2\theta-1\)のθを\(\frac{x}{2}\)に置き換えると
$$cos\ x=2cos^2\frac{x}{2}-1$$
\(\frac{1-tan^2\frac{x}{2}}{1+tan^2\frac{x}{2}}\)
$$=2cos^2\frac{x}{2}-1=cos\ x$$
検索しても計算過程が見つからない場合
検索しても計算過程が見つからない場合ココナラ
![]() を利用してみてはいかがでしょうか。
を利用してみてはいかがでしょうか。
ココナラ 登録方法
会員登録しなくてもサービスの検索はできます。
サービスの購入・出品には会員登録が必要です。
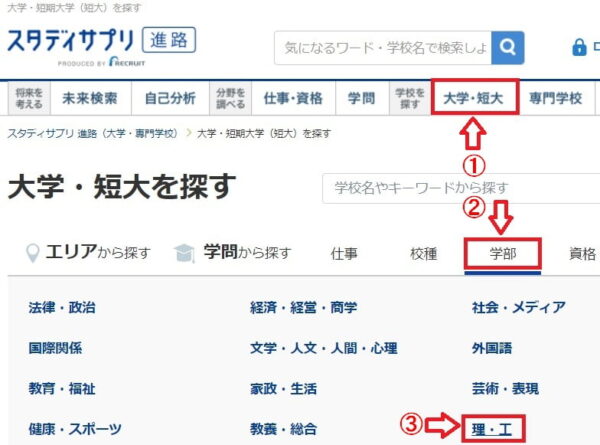
スタディサプリ進路 社会人向け の 使い方
スタディサプリ進路 社会人向けで社会人が数学を学べる大学を検索してみます。
大学数学入門(高校数学の復習)
- (1-tan^2(x/2))/(1+tan^2(x/2))の解き方 倍角の公式
- (1/2)log((1-cosx)/(1+cosx))からlog tan(x/2)を導く
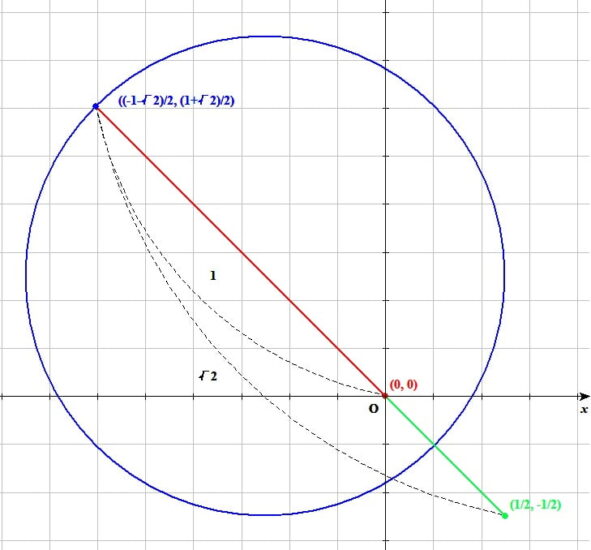
- 円(x-(2/7))^2+(y+(2/7))^2=(1/7)^2と直線y=-xの交点の座標
- 根号を含む線分の比